Does a Motor really Consume Energy?

This has been on my mind for quite sometime now but I think it's a question worth examining. We all know by now that it is absolutely impossible to achieve more energy out of a system than what went into it, especially if it's a closed system.

I have also posed this question on the physics.stackexchange.com website as "Why does Work Appear to be More than 100% Energy in a System?". The question doesn't seem to be going over very well over there and it may even get deleted because apparently nobody understood the question.

Here is an image of the message I received from them.

And here is a video of the question posed on that website :

It is claimed that it was made unclear even though I reworded it several times in order to make it as clear as I possibly could. In fact it's so clear that a second grader could understand it. So if that link eventually disappears then that just tells you what kind of character that website has deleting a completely honest and valid question. With that said we will continue the discussion right here.

For those of you who don't know the meaning of energy, work and power, I will give a quick explanation. This is necessary in order to understand the question and for those who already know this you can skip ahead if you want.

Energy is the Potential to do work, while work is just the energy put into motion or aka Kinetic Energy. Kinetic energy leads to power. Power of course is just the measurement of work, how long it takes, voltage, current, wattage and so forth.

Energy is measured in joules. One joule of energy is exactly equal to a One Watt Second. And power of course is measured in wattage.

If you had a capacitor with 1 joule of energy stored in it and then powered a small toy motor from it, how much work could you get out of the motor? Some may say well you would get 1 joule's worth of work of course. This is actually a fact, you do indeed power the motor with a joule of energy. Another way of looking at it is that the motor is powered with a watt of power for exactly one second. So this is a fine example of transferring energy in order to make it work with real flowing power.

It's easy to power a small motor with a mere 1 joule of energy until it's dead. We do it all the time, killing power supplies and then going out and then dishing out the cash just to do it all over again.

But, here's the kicker. If we recycle the energy several times, then we can multiply the amount of work the motor does to well over the original amount of 100%. What!? Yes that's right we can multiply the amount of work well past the 100% mark. Let me remind you though we are talking about work done here, not energy. But wait a second! We just got done saying that 1 joule of energy is equivalent to the power the motor used.

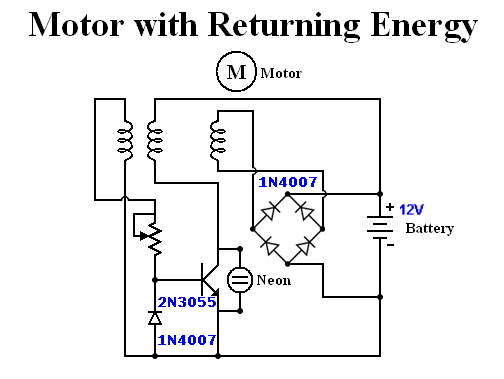
Here's a circuit diagram of a simplified pulse motor. Build it, it works. It is a trifilar coil, meaning it has 3 wires that are wrapped together. I'm not going into all the details of it's operation as that's a completely different topic all together. Basically there's a power wire, a trigger wire (for the transistor switching), and a pickup wire.

The pickup wire is just to separate the returned energy out of the circuit current flow. This is to prevent the battery from being shorted out in order to return the energy back to the battery.

Energy is stored in the power wire which is induced into the rest of the wires. It's stored as a magnetic field around the coil of wires. During the pulse off phase when the current is shut off or the power is disconnected the magnetic field collapses. It has to go somewhere so usually this ends up being a high voltage spike. This is the energy that is being returned to us and that we can recycle and reuse again.

Later on in another post I will explain a little bit about the collapsing electromagnetic field. I will also explain why the voltage is so high coming out of a collapsing magnetic field. It has to do with the near infinite speed of the returning energy.

In another words, it is perfectly possible to obtain more than 100% power, but not more than 100% energy. Really? How is that possible if power comes from energy and is even equivalent to energy in the first place?

Let's think about this for a second shall we? For example, let's say we have a capacitor charged with just 1 joule of energy powering a small toy motor. There's a meter in between the motor and the capacitor. Let's say the meter is between the negative of the capacitor and the motor on the negative side measuring current. Whatever current the meter reads coming out of the capacitor will be the exact same current going into the motor. Let's say it's reading 1 amp coming out of the capacitor and 1 amp going into the motor.

A meter sitting across the capacitor will read let's say 1V. A meter sitting likewise across the motor will read the exact same 1V. So it's safe to say that 1 Watt of power is coming out of the capacitor while 1 Watt is going into the motor. This seems like a 100% transfer of energy going on doesn't? Well it is.

I would like to make a clear distinction between transferring 100% of the energy and that none of that energy is recoverable. In fact 0% of the energy is unrecoverable if we don't do anything about it.

However if we transferred this energy one time and one time only, then we can only power the motor once with the same energy. For example the capacitor is completely drained until the motor has no more power left to run. But if we recycle and reuse the energy repeatedly then things start to get a little bit more interesting. Here is where the reality shift in the matrix begins to occur.

Now not only can we power the motor with 100% of the energy, but we can power the motor again, even by another 100% or more by using the same energy over again! Now the energy of the system, (or at least the capacitor) doesn't increase above 100%, for that's impossible. But the amount of work done on the motor does increase, by several magnitudes of order. This is not impossible.

So in another words, if we repeatedly reuse and recycle the same 100% energy over and over again, then we can multiply the amount of work done. We can get more work out of the system than what we originally would have been able to do if we were to use the energy only once.

So what does this have to do with a motor consuming or not consuming energy? Let's take this example for instance. Let's say we have a gallon of water and we pour out the water over a paddle wheel. Spinning mass stores energy. The paddle wheel is now spinning and doing work. If we let the water pour over the wheel and let it splatter all over the floor, then it's completely wasted away. We then have to go get more water.

Now take that same bucket of water and fill it up again and pour it over the wheel again. This time we place another empty gallon underneath the paddle wheel and capture all the water as it passes over the paddle wheel, thereby saving all of it. The water represents energy and the gallon of water represents the capacitor.

As you can see if we keep raising the water that's already fallen over the wheel we can get more work out of the paddle wheel without increasing the amount of water. Apparently the water or (energy) has nothing to do with the (power) produced at the wheel. Although the water has been converted from potential to kinetic energy, the kinetic energy can increase without increasing the potential energy.

This is how we recycle energy in order to continually use it over and over again. We were able to move the water around and make it do work on a paddle wheel, did the paddle wheel consume the water? No. So yes we can make electricity do work without really consuming any of it, just as long as we recycle it for further use and further work.

If we use 1 joule of energy only once, then we will only get one joule's worth of work out of our little toy motor. But if we use 1 joule of energy over and over again then we can get more work out of the motor than if we only used it once.

The fact that 100% of the energy that the system started out with has passed through the motor and has been transferred through the motor presents a bit of a paradox. How can the motor receive 100% of the energy, which of course converts it into 100% of the power, more than once?

Let's examine the question a little bit closer. The energy is stuck in a closed loop. It's a loop, a circle. Electricity comes out the negative side of a battery then travels into one side of the motor then comes out the other side of the motor and then back into the positive of the battery. It does all this in order to kill itself. This loop of current coming out of the negative of the battery only becomes a current the very instant it realizes that the (information) of the positive exists.

The very instant the positive receives back this loop of current is the very instant that the battery or whatever power source it is begins to kill itself. At least the current of power and energy is doing some kind of useful work before it kills itself.

So in a sense the energy is completely wasted away because we aren't saving any of it in an electrical sense. If the load is a motor, in fact the energy is being stored in the motor's magnetic field. Normally we don't get the energy stored in the magnetic field returned back to us.

Take a light bulb for instance, we most definitely aren't storing the energy in anyway at all as the energy is completely wasted away as light and heat. Again though at least we are getting some kind of useful work out of it as it's in the process of being wasted away.

So in a sense we are wasting away the energy by not keeping it or storing any of it in any way at all electrically wise. But we aren't wasting it completely away either because we are getting useful work out of it in the form of light and heat or a spinning motor. But all at the expense of destroying the power source. In another words we have to destroy the power source in exchange for getting useful work out of our loads.

This is the paradox. Should we look at this simple closed loop system only as input energy only? Where's the output? Like mentioned earlier current comes out of the negative of a battery, is this output? That same current also goes back into the positive of the battery, is this input? Here's a case where the output kills itself by going back into itself in order to destroy itself.

Now place a load in between that circle of destruction and now we can at least get useful work out of that circle of death. The other part of that paradox is that all 100% of the energy that's going to it's death is indeed passing through the load as well.

So the load is getting 100% of the energy passed and transferred through it. If we refer back to the 1 joule of energy stored in a capacitor then this represents the motor being powered with 1 watt of power for one second.

This 1 watt of power came from 1 joule of energy and it's exactly proportional and equal to each other. If we completely deplete the 1 joule of energy, then we have 0% energy left. It has completely destroyed itself, but at least we got one watts worth or 1 joules worth of work out of it right?

So now let's take a look at recycling the energy. We can never get over 100% energy in a system because of the conservation of energy law. However we can take that 100% energy and recycle a portion of it electrically speaking. How can we completely drain 100% energy down to 0% and still be able to collect a certain percentage of it?

It's almost as if the energy has performed a disappearing act. When in fact it has performed a disappearing act and then magically it appears somewhere else. I will explain this further here in a moment.

Let's say we recycle the energy through the use of the motor's magnetic field when it collapses. It's the only way to recycle energy electrically. We started out with 100% of the energy and then ended up with 0% of the energy. We made the energy pass through a motor and did 100% work. Yet simultaneously we saved or stored let's say 80% of the energy, are these two things really the same thing or are they completely separate from one another?

In another words have we simply transferred the 100% energy from point A to point B? Or has 100% of the energy gone to destroying the power source and transformed into heat while still collecting 80%? If that were true then we would actually have 180% not just 80% energy left over.

I mean think about it. A capacitor or battery destroys itself but not the energy. Energy can not be created nor destroyed. It's impossible to create or destroy energy but we can transform it. Ok so the energy was completely wasted away inside of the power source as dissipated heat.

So the power supply basically experiences a complete heat death, however it's still energy albeit completely wasted away as heat but it's still energy nevertheless.

The work of the motor is equal to the amount of energy that we started out with namely 100%. If we recycle 80% of the energy how is it then that we are able to do 80% more work on the motor then? Would this constitute 180% energy in the system?

Yes it's true that 80% is just part of the 100% that we started out with, yet we are able to make the motor run with 180% power. That's 1.80 Joules of energy required in order to power the motor with 1.80 watts of power.

There is only one explanation of the 80% recycled energy that has been returned back to us. That is it has nothing to do with nor is part of the original 100% energy that we started with.

Are you beginning to see the paradox in which I speak of yet? If we start out with 1 joule of energy and make it do the work of 1 watt of power for exactly 1 second then we have utilized all 100% of the energy by making it do 100% of the work. Wouldn't we need more than 1 joule of energy in order to make the motor run with more than 1 watt of power for 1 second?

How is the motor running with more than 1 watt for 1 second? How is the motor running with more than 1 joule when we started out with just 1 joule of energy?

Yes it's true we are recycling the energy at a percentage that is less than 100%. Yet we are able to get more power which is supposed to be equivalent and proportional to the energy. Does this make sense to you? How can that be? How can we have 180% energy in the system? Is the 80% part of the 100% or is the 80% actually completely separate from the 100% and all on it's own?

Have we simply transferred the 100% and recycled 80% from that very same 100%? Or is the 80% energy in addition to the 100% energy that we started off with?

Perhaps if we consider the forward current first. This forward current represents the transfer of power into the motor. Simultaneously around this forward current there is a magnetic field. How can we have a forward current causing power to occur while simultaneously storing energy in it's magnetic field? Is this really additional energy? Is this really where the secret lies?

This forward current performs 1 watt of power for one second on the motor. This is power. The power came from 1 joule of energy. Now let's consider the back current. This back current can give us back 80% of the energy that we started with and it comes from the magnetic field that has already collapsed. Again, is this really an additional 80% energy if the forward current of 100% energy has already occurred?

Let's take our microscope and zoom in even further on the situation. The energy coming from our power source is going back to basically kill itself right? Energy can't be created nor destroyed, it's more like a heat death inside of the power source. It's not like the energy is being destroyed, it's just being completely wasted away as heat radiating away inside of the power source. Same thing for our example of the gallon of water and letting it splatter all over the floor or ground.

Ok so let's say we wasted 100% of our energy away as a heat death inside of the power source. The forward current went through the load and now the power source is completely dead, 0% energy left. This forward current has a magnetic field around it. It is said that this magnetic field is in exact proportion to the 100% that we completely wasted away inside the power source. For the most part we can recover part of that 100% from the magnetic field collapsing.

Let's say the system was perfect. In a perfect world in an ideal system of 100% we could recover 100% of the energy. We would do this through the use of a collapsing magnetic field which would be proportional to the heat death or wasting away of the energy inside of the power source. Let me say something right here. First of all, all 100% of the energy that went into destroying the power source is still there! It's just now in the form of heat that destroyed the battery and escaped out into the atmosphere.

What is this guy talking about you may ask yourself??!! It's really easy to understand. We measure how much power or wattage that is coming out of a battery or capacitor. What we are really reading is the heat death occurring in the power supply. We are just measuring the amount of destruction of the power source. We are also measuring the amount of energy that is being transformed into heat inside of the power source.

It no longer is any use to us because it's in a form that is no longer electrically accessible. However it's still 100% of the energy regardless. In a sense we can say that 100% of the energy still exists, so then what does that make the magnetic field that surrounds that 100% energy? Remember we are still speaking about the forward current that is going back to the battery to be completely wasted away, it still exists!

So if we have 100% of the energy that still exists, then what in the heck is the energy contained in the magnetic field that we can get returned back to us? This returned energy is obviously more than the 100% energy that went into being wasted away inside the power source!

But since this isn't a perfect world then that's impossible. It would require an environment without resistance. If we were actually able to do this then what about the energy left over from the magnetic field pushing on another magnetic field on a rotor? Hopefully by now we understand that spinning mass stores energy. I speak of this concept in my original article Motor with Returning Energy.

What about the example with the gallon of water? Even though a gallon of water can't fully be compared to an electromagnetic system, it's just a representation to try to make it more clear as to what is actually happening. It's not a perfect example, so I'm sure I might have left something out of it.

If we take our gallon of water and pour it over a paddle wheel and collect the water into another gallon then we could actually store all of our energy and still have energy left over from the spinning paddle wheel. But let's take a look at that analogy from the very beginning.

The water itself would represent the energy of the system that is now moving from point A to point B also known as power.

It would take energy and power in order to lift the gallon back up to the point in order to pour the water back out again. Let's say it takes 1 watt of power to do that. Ok so now gravity takes over and pulls the water back down and over the paddle wheel. So now we have the 1 watt of power returned back to us.

The difference in between the height of the gallon of water and the ground would represent the potential difference or voltage.

The water represents energy but it takes work to move that energy around. If we started from the point where the water is already raised to a certain height, then this would certainly appear as free power and or energy. But it's not.

To have a fully functioning and complete system we would first have to raise the energy or water up to that certain height. This requires energy and power of course. We have to raise the potential if we want to get a power flow of current right?

How much power would the paddle wheel generate? Does it have anything to do with the amount of power it takes to lift the water back up to the same point again? Or does it have anything to do with the actual current flow of water or energy?

The amount of power required to lift the water back up again is work that has to be done against gravity. So it seems to be that the amount of water or mass that comes pouring down because of gravity and then collected, is the exact same amount required to pull the water back up against gravity.

Of course there's also the matter of some kinetic energy being transferred from the water to the paddle wheel. The paddle wheel takes away some of that energy and then stores some of it in it's kinetic rotation.

The energy or (water) has now moved from the state of potential to the state of kinetic. Has the water been destroyed from a heat death? No of course not, we have simply recycled it and collected it in another gallon. The amount of work it takes to move the energy around from low to high and back to low again would be identical but it's not because the paddle wheel is inserted into the system.

Some of the energy has departed into the paddle wheel thus storing it. We would have to rely on the paddle wheel to generate power. The power would then give us back the energy required to lift the gallon of water back up to the same point it started from. This would be required in order to allow gravity to pour the water back over the wheel again. Thus continuing the cycle.

We didn't have to get rid of any of the energy in the system in order to do this. Just because water or (energy) is flowing and causing power to occur multiple times doesn't mean that it's being depleted or seemingly disappearing and wasted away, at least in a perfect system it doesn't.

In summary the example of the water wheel would go something like this. Let's say it takes one watt to lift the gallon of water to a certain height. Ok now gravity takes it back and the same one watt is transferred into the paddle wheel, meanwhile we are collecting the same amount of water, or mass (energy).

This is an example of energy performing work without being depleted. However in order to lift the water back up again requires 1 watt. So we would have to rely on the generation of one watt being stored in the paddle wheel. If that' the case then we are left in a perfect system of 100%. In a perfect world, that sounds about right.

But a system of water being moved around past a paddle wheel is quite different from a system of electricity and magnetism, or is it? Let's compare the two examples together. We start with 1 joule of energy in a capacitor. This is 1 gallon of water. We then power a motor with this 1 joule of energy and cause 1 watt of power to flow through the motor. The energy has now moved from potential to kinetic.

This would be similar to letting gravity take over and pouring out the water over a paddle wheel. We now harness the energy stored in the motor's magnetic field and get back oh let's say a mere 50% of the energy. This would be like saying that we let the water fall over the paddle wheel and collect only half of it because half of the water fell out of the system and onto the ground while the other half is collected in another gallon.

In a nutshell we can take energy or the potential to do work and move it around. This causes work and power to occur without depleting the energy. Except of course losses incurred from resistance is the only thing that really depletes energy.

We can move this energy around multiple times and cause multiple instances of power flows to occur. We are simply causing energy to keep flowing repeatedly through a load without depleting the energy too much. The real question is, how are the multiple instances of power, which increases beyond the original 100%, related to the energy that remains at or below 100%?

I suppose at this point it would make sense to say that potential energy is completely different from kinetic energy yet very similar and related to each other. Are they part of one another or are they completely separate and individual from each other?

It seems relatively simple at first but when examined more closely we see that we can take potential energy which does not multiply and cause kinetic energy to occur which does multiply. How can kinetic energy multiply from a potential energy source that does not multiply and remains the same?

Now I would like to finish up with a summary with all the factors combined together that I would like to emphasize here. A capacitor or battery starts with 100% of the energy in a system. A forward current that comes out of a battery or capacitor and or through a load and then back into itself is completely wasted away. But that doesn't mean that the energy has disappeared! The energy is still there except now it has simply been transformed into a form of heat inside the power source.

It is still 100% of the energy. Now take into account that there is yet even more energy stored in the magnetic field from the forward current. You now cut off the current from flowing and being transformed into heat inside the power source which is still 100%. It's still the same energy but it's just in another form now.

Now the energy that has now been transformed into heat has stopped flowing. This causes the magnetic field to collapse and return let's say 80% of the energy. Now we literally have 180% of the energy left in the system.

One hundred percent is in the form of heat returning back to the environment never to be electrically utilized ever again. While the other percentage is 80% from the collapsing magnetic field which can be electrically utilized several times over.

These two energies have nothing to do with each other and are completely separate from one another.

What we can't see is the form of energy it's been transformed into which is heat. However we can measure the product of this wastage on meters as electrical power, voltage, current and wattage.

This all occurs with the forward current. Meanwhile the back current is still giving us even more energy. This is the energy that we are also all familiar with on our meters and it came from the collapsing magnetic field.

What does this tell us about the magnetic field of the forward current? Logically speaking and thinking in this thought experiment, it means that the magnetic field is a stand alone energy source that is individual and separate from the current in which it comes from. This is evidenced as 100% of the energy in the form of heat co-existing along with the energy in the magnetic field. We can measure this forward current with a meter.

This may also explain why we are able to power a motor with multiple instances of power in the form of wattage, that we can also measure on a meter. We can do all that with just a starting energy of a mere 100% stored in a battery or a capacitor.

The multiplied power of the motor could also be successfully converted or inverted into electrical power without any ill consequences. Then we would be able to convert that power back into even more reusable energy. We have already equated the power coursing through the motor as being equivalent and proportional to the energy source from whence it originally came from.

Three places that energy exists in

• 100% of the energy in the system is transformed into heat inside the power source and is completely wasted away. This wasted energy is the forward current powering a motor.
• In a perfect system we could get all 100% of the energy returned back to us from the collapsing magnetic field in the form of a back current, that's another 100% energy
• A spinning rotor is an additional energy source in which it is locked up inside the kinetic spinning mass, and is not easily accessible. The amount of energy obtained in this manner is variable.
• Not including the spinning mass of the rotor, a total amount of energy that is most definitely contained in a spinning motor system is 200%.

If you agree or disagree please leave any of your questions and comments down below as they are welcome.